Question
Here is the question : FIND THE VOLUME USING V = 1/3(L X W X H), WHERE L = 2, W = 3, AND H = 4.
Option
Here is the option for the question :
- 8
- 16
- 24
- 84
The Answer:
And, the answer for the the question is :
Explanation:
Use the formula volume = 1/3(length x width x height) to determine the volume of a rectangular pyramid. Then, finish the expression v = 1/3(2 x 3 x 4), where v is a variable. This equation can be reduced to v = 1/3 x 24, or v = 24/3, yielding v = 8 even without the aid of a calculator.
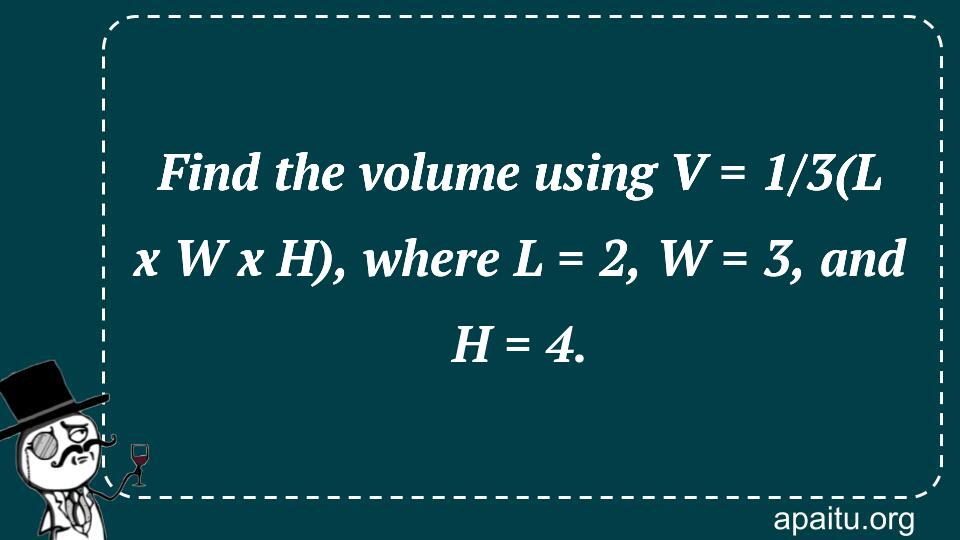
Calculating the volume of an object is a fundamental concept in mathematics and physics. The formula to find the volume of a three-dimensional shape is V = 1/3(L x W x H), where L represents the length, W represents the width, and H represents the height. In this article, we will use this formula to find the volume of a specific object given its dimensions.
Let’s consider an object with a length of 2 units, a width of 3 units, and a height of 4 units. Using the formula, we can calculate the volume of this object as follows:
V = 1/3(2 x 3 x 4)
V = 1/3(24)
V = 8
Therefore, the volume of this object is 8 cubic units.
It is worth noting that the formula we used to calculate the volume of this object is only applicable to certain types of three-dimensional shapes. Specifically, it is suitable for calculating the volume of a pyramid, which has a polygonal base and triangular faces that meet at a common vertex. In this case, the length, width, and height represent the dimensions of the base and the height of the pyramid.
For other types of three-dimensional shapes, such as cubes, spheres, and cylinders, different formulas are required to calculate their volumes. For instance, the formula for the volume of a cube is V = s^3, where s represents the length of one side of the cube. Theformula for the volume of a sphere is V = 4/3πr^3, where r represents the radius of the sphere. The formula for the volume of a cylinder is V = πr^2h, where r represents the radius of the base and h represents the height of the cylinder.
Knowing the formula for calculating the volume of a specific object is essential in many fields, including architecture, engineering, and physics. For example, architects and engineers need to calculate the volume of building materials, such as concrete and steel, to ensure they have enough materials for construction projects. In physics, calculating the volume of an object can help determine its density, which is an important property in many scientific experiments.
there are also other ways to visualize and understand the concept of volume. One way is to think of volume as the amount of space an object takes up in three dimensions. For example, imagine filling a container with water. The volume of the water is the amount of space it occupies within the container.
Another way to understand volume is to think of it as the capacity of an object. For instance, the volume of a cup is the amount of liquid it can hold. In this case, the shape of the cup determines its volume, which can be calculated using the appropriate formula.
calculating the volume of an object is a fundamental concept in mathematics and physics. The formula for calculating the volume of a pyramidis V = 1/3(L x W x H), where L represents the length, W represents the width, and H represents the height. By using this formula, we can easily find the volume of a pyramid