Question
Here is the question : IF X + 7 = 10, AND 2X + 11 = Y, WHAT IS THE VALUE OF Y?
Option
Here is the option for the question :
- 4
- 9
- 17
- 29
The Answer:
And, the answer for the the question is :
Explanation:
The solution to this problem requires a few steps to complete. To begin finding the solution for x, start by subtracting 7 from 10 to get the answer x = 3. You may utilize the information we just went through to figure out what y is now that we know x equals 3. To begin, we will obtain the number 6 by multiplying 2 by 3. The end result for the value of y is going to be the number 17, which can be found by adding the numbers 6 and 11.
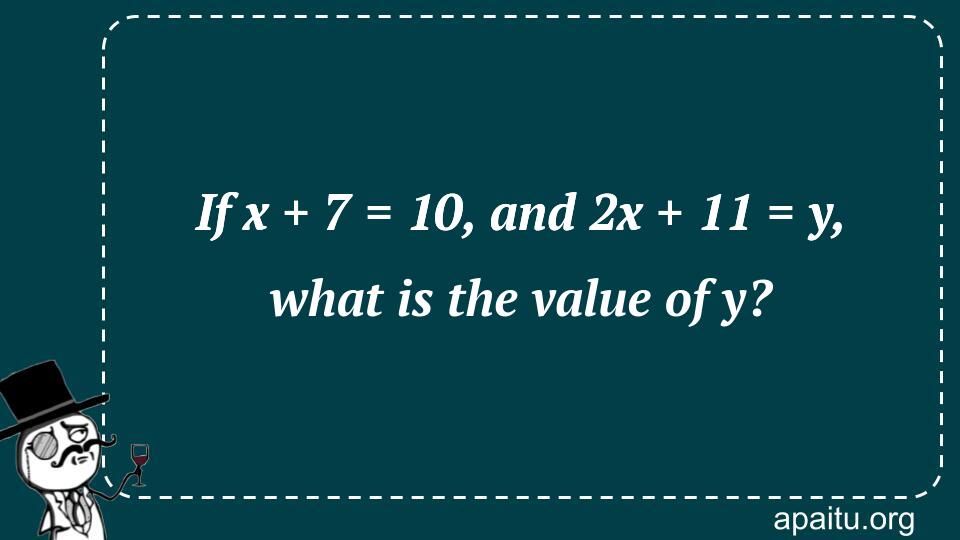
In mathematics, it is common to encounter systems of equations that involve multiple variables. Solving these equations involves using algebraic techniques to isolate the variables and find their values. In this case, we are given two equations and asked to find the value of the variable y.
The first equation is x + 7 = 10. To solve for x, we can subtract 7 from both sides of the equation, giving us:
x = 3
The second equation is 2x + 11 = y. We can substitute the value of x that we just found into this equation, giving us:
2(3) + 11 = y
Simplifying this expression, we get:
6 + 11 = y
Therefore, the value of y is:
y = 17
It is important to note that when solving equations, we need to follow the rules of algebra and the order of operations. In this case, we first solved for x by isolating it on one side of the equation. Then, we substituted the value of x into the second equation to find the value of y. By simplifying the expression, we obtained the answer of y = 17.
Furthermore, it is important to understand the concept of variables in mathematics. A variable is a symbol that represents a value or quantity that can change or vary. In this case, x and y are variables that can take on different values depending on the context of the problem. By using variables, we can write equations and expressions that can be easily adapted to different situations and scenarios.
solving systems of equations involves using algebraic techniques to isolate the variables and find their values. In this case, we were given two equations and asked to find the value of y. By solving for x in the first equation and substituting that value into the second equation, we were able to simplify and obtain the answer of y = 17. It is important to follow the rules of algebra and understand the concept of variables in order to successfully solve equations and problems in mathematics.